Abstract
For decades, recovery of ore at the Quémont Pillar in Rouyn-Noranda, Québec was relatively impossible due to the nature of very soft, high water content clay comprising the overburden material above the abandoned mine. Following the success of other ground freezing projects in the area, an ambitious scenario was proposed which would result in the largest ever frozen ground excavation in North America. The field and laboratory investigations are described as well as the engineering analyses used in the design of the 61-meter diameter, 35-meter deep frozen earth cofferdam. These analyses include interpretation of the laboratory data, conventional and numeric structural evaluation and time-dependent thermal finite element modeling. A brief discussion of the excavation process is included.1. Background
The Quémont Mine in Rouyn-Noranda, Québec, Canada located approximately 389 miles (626 km) northeast of Toronto was completed and closed several decades ago. A crown pillar remained in place and was known to contain approximately 11000 m3 (15000 cubic yard) of zinc. The deposit was located 80 to 120 feet (24 to 37 m) below water-bearing, unconsolidated mine tailings and very soft clay. Mining from the surface had been considered for several years; however, excavation support was always considered to be the limiting factor, both technically and economically. In 1997 the concept of using ground freezing was considered. The initial approach was a very large groundwater barrier, which would enable dewatering and open cut with very shallow slopes. This approach, while technically appropriate, was too expensive considering the limited amount of ore available for mining. The concept of creating a large, structural frozen shaft was also evaluated. While this approach required considerably fewer freeze pipes, the frozen ground would have to function as a structural wall in addition to providing groundwater cutoff. The selected approach was to create a 200-foot (61 m) diameter excavation in an area of the mine, where the overburden soils ranged from approximately 70 to 100 feet (21 to 30 m) depth. To the authors’ knowledge, there have not been any frozen earth structures of this size completed in North America and this project would be a technical milestone in the freezing industry. Before ground freezing could be fully evaluated, it was necessary to first complete a preliminary design and cost estimate to ensure overall project feasibility. Key components of the ground freezing design included: Total thickness of the frozen earth wall, number of freeze pipes required for wall formation, refrigeration capacity, the length of time the structure could remain open without substantial creep deformation and resulting structural failure. Before any of these design components could be addressed, it was necessary to gain additional information through field borings and laboratory tests.2. Field Investigation
While there were several exploratory borings related to the ore-bearing rock, there was limited information available on the overburden soils. An exploratory drilling program was conducted. The purpose of the drilling program was to define the top of the bedrock with four sampled borings 90 degrees apart around the excavation perimeter and to recover relatively undisturbed tube samples for strength testing. Due to the relatively soft nature of the clay and the presumed high water content, frozen soil compression tests would be required to determine the long-term strength of the frozen clay as well as the elastic moduli needed in the structural analysis. Four borings were completed around the perimeter of the proposed shaft at the locations illustrated in Fig. 1. The borings were completed with a Foremost Barber rig utilizing reverse rotary air circulation. Sampling the drilling cuttings returning in the exhaust cyclone completed continuous soil classification. At those depths where soft clays were observed, a 3-inch (7.6 cm) diameter sampler was slowly pushed into the formation. Immediately after extraction of the sample from the boring, and removal of the six-inch (15.2 cm) liner from the sampler, plastic end caps were applied and sealed with water-proof tape. The samples were then transported via ground from the job site to a frozen soil testing laboratory in Milwaukee, Wisconsin. The trip took approximately 14 hours and the samples were subjected to considerable vibration. It is also possible, but not confirmed that the samples may have been exposed to sub-freezing temperatures for a short period of time. When the samples were opened in the laboratory, they were in semi-liquid state. It is uncertain that the liquefaction was from vibrations during shipment (indicating this could be a sensitive clay) or from the effects of being subjected to colder temperatures and possible freezing and thawing. Regardless of the cause, the samples were too disturbed and tests were discarded. It was then necessary to complete another test boring for the purpose of recovering samples of the soft clay.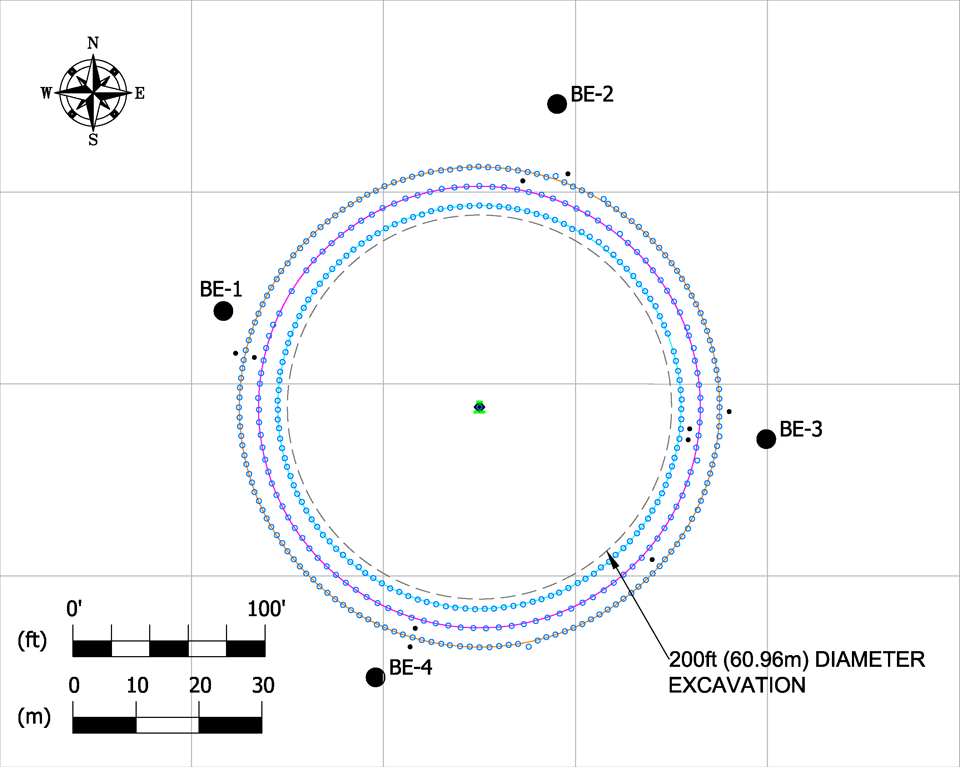
Fig. 1: Site Plan
A drilling rig was once again mobilized and conducted additional borings. Soil profile information from the previous borings was used to identify the clay strata from which samples would be retrieved for frozen soil compression testing. In order to minimize the disturbance from vibrations during transportation the samples were frozen immediately after extraction from the sampler. The six-inch (15 cm) long samples were sealed on one end and wrapped with an one-inch (2.5 cm) foam insulation jacket. One end of the sample was left exposed to allow pore water to escape during the freezing process. The samples were placed in a freezer on site and allowed to freeze for 24 hours. Once the samples were frozen, the exposed end was sealed and the samples placed in a cooler with dry ice. The samples remained frozen for the shipment to the laboratory where upon arrival, they were placed in a freezer until tested. Review of the drilling logs concluded that there were four distinct soil strata as presented in Table 1. Fig. 2 presents an approximate profile of the subsurface strata. It was observed early in the investigation that there was no simple way to define the depths of the strata. The engineering design for projects considered the properties and behaviors of all four strata; however the Stratum II Clay presented the most difficult challenges in completing this project. For brevity of this paper, only Strata II and III are discussed in greater detail.| Stratum | Description |
|---|---|
| I | Tailings |
| II | Clay |
| III | Sand and Gravel |
| IV | Bedrock |
Table 1: Preliminary Strata Classifications
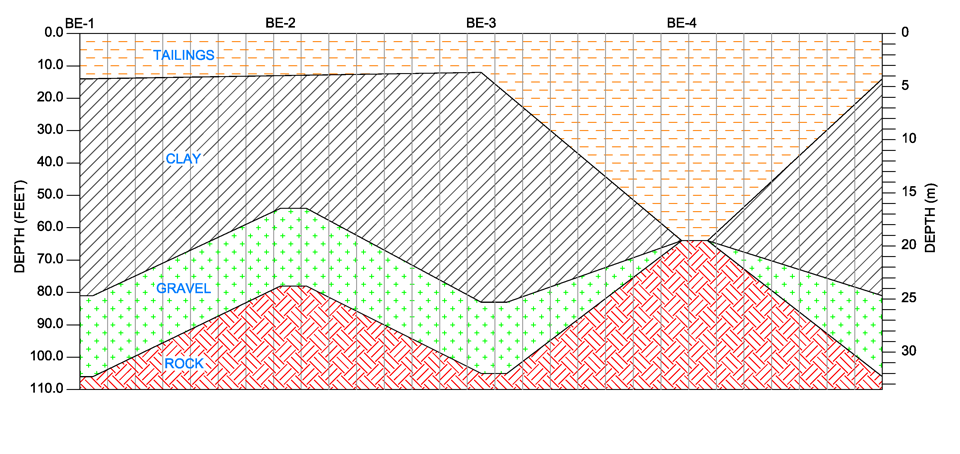
Fig. 2: Subsurface Strata
High groundwater velocity is one of the most common causes of problems when trying to form a frozen earth wall. High permeability, coupled with a groundwater gradient that produces a velocity of greater than three feet per day (1 m/day) will retard or even prevent freezing by introducing more heat into the ground than the freezing system can extract. Field inspection during the test boring process concluded that Stratum III, the sand and gravel, were pervious enough to introduce a potential problem if a gradient existed. Each of the four boreholes was finished with a piezometer that was screened through the entire thickness of Stratum III. After finishing the development of the piezometers, groundwater levels were recorded and found to be near the ground surface. The level was essentially equal in all four piezometers indicating that no gradient existed across the site and thus no indication of a groundwater velocity greater than 3 feet per day (1 m/day).3. Laboratory Testing
While the hydraulic properties of Stratum III were evaluated in the field, the soft, high water content clay of Stratum II caused concern for both frozen strength and potential deformation. The large diameter of this excavation would result in stresses significantly higher than those experienced in smaller, deeper shafts that are commonly frozen in the mining and civil construction industry. The frozen soil laboratory testing was a two-phased approach. Unconfined, constant strain rate tests were conducted to determine the elastic modulus of the frozen soil and unconfined, constant stress compression tests were conducted to evaluate long-term creep strength and deformation. Sample preparation for both tests was identical. The samples remained in the freezer from the field until preparation for testing, in the original brass sample liner and the insulation jacket. Following extraction and trimming, two clay samples were tested in the constant strain rate frozen compression test. The constant strain rate, unconfined compression tests were conducted using an electric pulse generator that was calibrated for strain rates for the particular compression device used in this testing. The rate selected was 0.0046 inches per minute (0.0018 cm/minute) or approximately 2 percent strain per minute.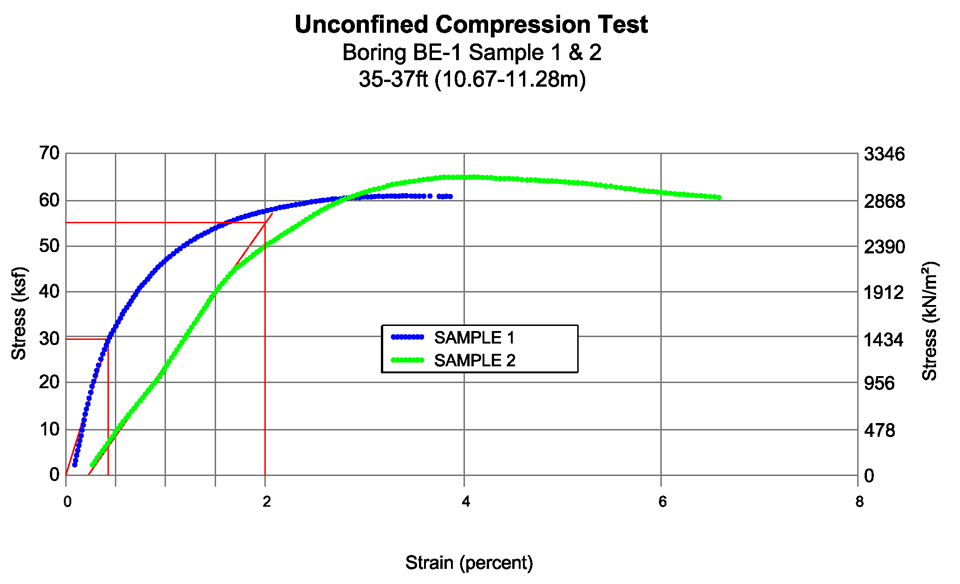
Fig. 3: Unconfined Compression Tests
Two samples of the clay, taken from boring BE-1 at a depth of 35.0 to 37.0 feet (10.67-11.28 m), were tested. The test temperature was -10 oC (14°F) and both test yielded unconfined compression strength of approximately 60 ksf (2930 kN/m2). Fig. 3 presents the two stress versus strain curves of these tests. These tests were used to approximate the stress levels to be applied in the creep tests as well as to evaluate the elastic moduli of the frozen clay.![Figure 4: Unconfined Creep Test (30 ksf) [1465 kN/m2]](https://groundfreezing.com/wp-content/uploads/2012/04/image004.png "image004")
Figure 4: Unconfined Creep Test (30 ksf) [1465 kN/m2]
Creep tests were conducted to determine the long-term strength of the frozen soil. The creep testing apparatus applied a constant load to the piston on the triaxial cell using regulated compressed air. The load was measured by monitoring the pressure in the loading piston, while deformations were measured using the same transducer that was used in the constant strain rate test. Two tests were conducted at -10 oC (14 °F) with constant stresses of 30 and 55 ksf (1465 and 2686 kN/m2). The 30 ksf (1465 kN/m2) test failed at approximately 190 hours and 6% strain, while the 55 ksf (2686 kN/m2) test failed at 13 hours and 9% strain as illustrated in Fig. 4 and 5. While it would have been desirable to have at least one additional creep test to evaluate the parameters, it was not possible during the original evaluation. The additional test would have been at a lower stress level and would have required several weeks to complete. Using the laboratory testing program and sources cited in the references, a summary of the material property ranges are presented in Table 2.![Figure 5: Unconfined Creep Test (55 ksf) [2686 kN/m2]](https://groundfreezing.com/wp-content/uploads/2012/04/image005.png "image005")
Figure 5: Unconfined Creep Test (55 ksf) [2686 kN/m2]
| Stratum | I | II | III | IV | |
|---|---|---|---|---|---|
| Tailings | Clay | Sand & Gravel w/Clay | Rock | ||
| Description | Units | Range | Range | Range | Range |
| Saturated Density γs | PCF | 100–130 | 110–130 | 100–125 | 170–180 |
| g/cm³ | 1.60–2.08 | 1.76–2.08 | 1.60–200 | 2.70–2.90 | |
| Moisture Content w | % | 20–30 | 40–60 | 20–30 | — |
| Friction Angle φ | Degrees | 25–35 | 0–10 | 25–35 | — |
| Coefficient of Permeability k | gal/day/ft² | 2–200 | 10⁻¹–10⁻³ | 10¹–10⁴ | — |
| cm/sec | 10⁻²–10⁻⁴ | 10⁻⁵–10⁻⁷ | 10⁰–10⁻³ | — | |
Table 2: Ranges of the Material Properties
Using the properties presented in Table 2, structural and thermal analyses of the proposed frozen earth structure were conducted.4. Structural Analysis
The structural analysis of the proposed cofferdam was based on a complex system of applied loads due to the varying elevation of the bedrock surface and the deposition of tailings. During the preparation of the structural analysis, two key principles were adhered to. First, the geometry and related internal stresses of a frozen earth structure change continuously throughout its lifetime. Second, the mechanical properties of the frozen soil may be varied widely due to temperature changes within the frozen mass. Frozen earth behaves plastically, exhibiting time and temperature dependent rheology. Given the heterogeneity of the ground, the structural analysis could only be modeled with a multi-dimensional finite element model. Before constructing the model it was necessary to approximate dimensions of the frozen earth wall. This approximation was based on estimates that were determined using conventional elastic and creep equations. The high water content clays of Stratum II would govern the size of the frozen earth structure from both an initial loading and time dependent creep deformations. Initial evaluations of the creep tests were conducted to approximate the required frozen earth wall thickness and potential creep deformation. From the constant stress creep tests presented in Figures 4 and 5 the following parameters were interpreted:- , the pseudo-instantaneous plastic strain
- , the failure strain
- , the time to failure, and
- , the creep strain rate
| Test Stress (ksf / kN/m2) |  |  |  |  |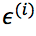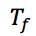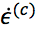
|---|---|---|---|---|
| 30 / 1465 | 0.3% | 6.3% | 180hrs | 5.6EE-6/min |
| 55 / 2686 | 0.7% | 4.0% | 6hrs | 9.2EE-5/min |
Table 3: Creep Test Parameters
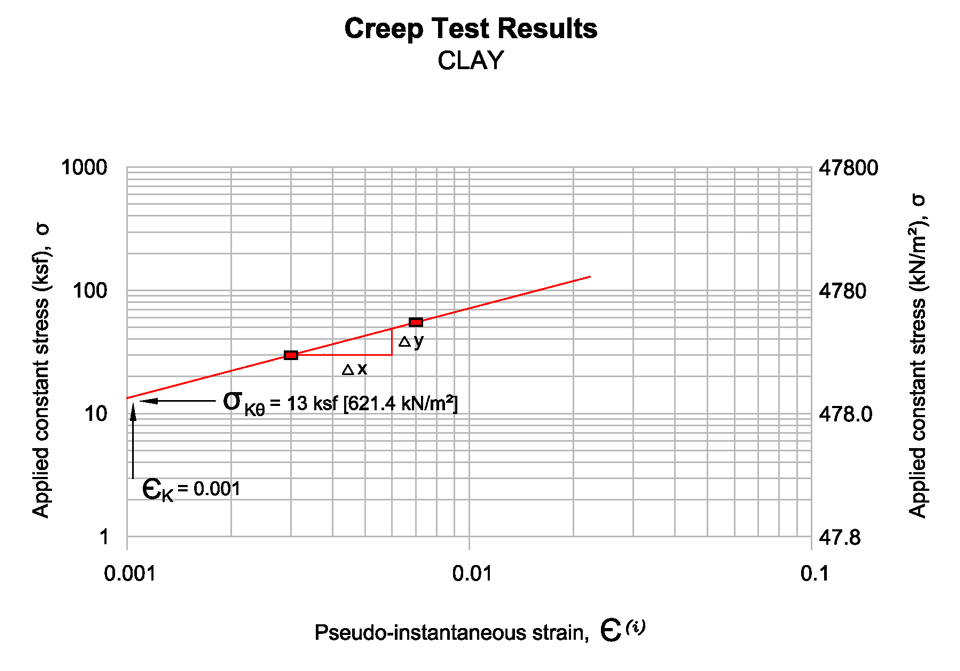
Fig. 6: Pseudo-instantaneous Strain vs. Applied Constant Stress
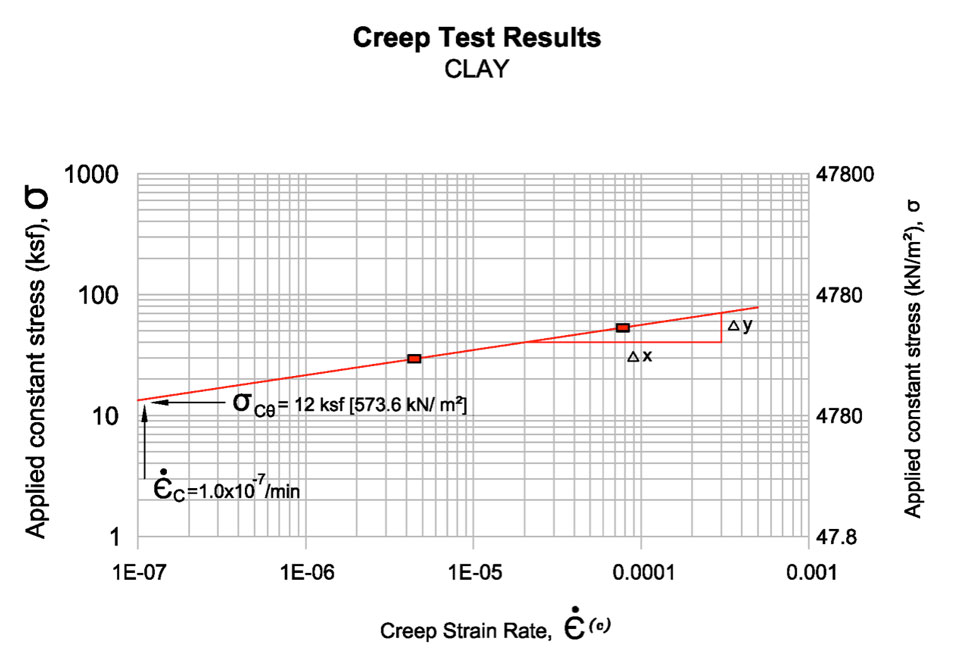
Fig. 7 Creep Strain Rate vs. Applied Constant Stress
Using the general creep equation
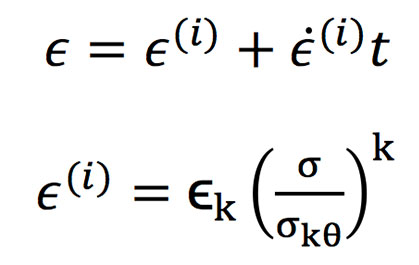
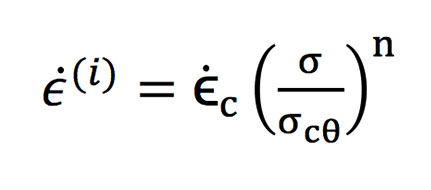From Fig. 6,
And from Fig. 7,
Substituting into the constitutive equation:
Using the constitutive equation and allowing a maximum strain of 5 percent, the stress versus creep age relationships were determined as presented in Table 4.
Where 
From Fig. 6,
And from Fig. 7,
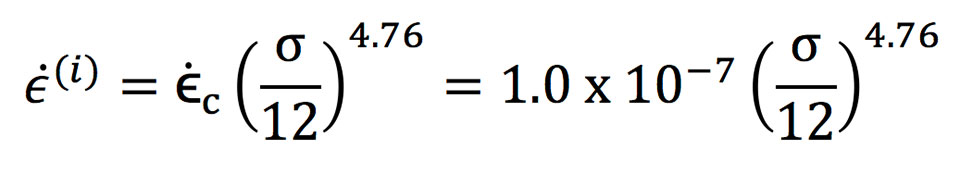Substituting into the constitutive equation:
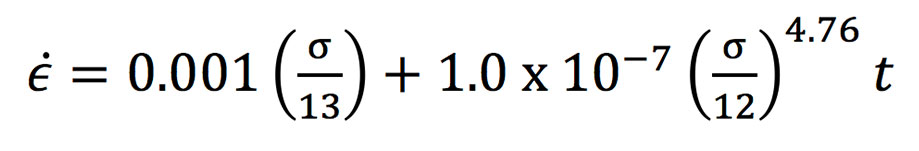
| Stress (ksf / kN/m²) | Creep Age |
|---|---|
| 27 / 1318 | 1 week |
| 23.4 / 1143 | 2 weeks |
| 20.0 / 977 | 1 month |
| 17.3 / 845 | 2 months |
| 15.8 / 771 | 3 months |
Table 4: Stress vs. Creep Age Relationship
Review of this information indicated that the service life of the proposed frozen earth cofferdam would be limited due to the creep characteristics of the frozen clay. Evaluation of the excavation, blasting and mining schedule indicated that the mining operation could be completed in three months. If the project would be successful, it was agreed that in order to maintain a safe excavation it would be necessary to limit the internal stresses to 15 ksf (733 kN/m²). Using active earth pressures and full hydrostatic load from the ground surface, internal stresses could be limited to 15 ksf (733 kN/m²) if the frozen earth wall were at least 30 feet (9.15 m) thick. While this approach was satisfactory for an estimate of the frozen wall thickness, it was based on a uniform cylinder assuming the entire structure was composed of the high water content frozen clay. Given the heterogeneity of the subsurface conditions, it was determined that the structural analysis could only be modeled with multi-dimensional finite element design. A purely elastic model was evaluated using the SAP 2000 program. The model was developed based on the information gathered from the field and laboratory investigation and solved for initial stresses and elastic deformation. The computed stresses were then compared to the mechanical behavior of the soil under sustained loading conditions. In other words, the stresses computed against time with the constitutive equation would be used as limits for both the allowable internal stresses with the frozen wall and the maximum time the excavation could be safely open. From Table 4, it was decided to limit the internal stresses of the frozen wall to 15.8 ksf (771 kN/m²) and use 3 months as the maximum time the excavation could remain open. The initial model was prepared using the generalized soil profile shown in Fig. 2. This cross section was divided into a series of discrete elements. Each element then had appropriate material properties assigned based on the information provided in Table 2. The individual shell elements were based on a 30-foot (9.1-m) wall thickness. The model radius and diameter was based on the center of the frozen wall and assumed a 200-foot (61-meter) diameter excavation. In accordance with the varying overburden stratification, three different lateral earth pressure loads were computed. These pressures were based on the material properties shown in Table 2 and assumed at-rest soil pressures. It should be mentioned that there will be some deformation within a short time following the initial excavation resulting in substantially lower active earth pressures, which could have been used in the analysis. However, it was the intention to minimize the internal stresses by increasing the frozen wall thickness therefore minimizing deformation, making the use of at-rest pressures a conservative approach. The execution of the FEM model yielded internal stresses within the clay layers of 13 ksf ( 621kN/m²). As anticipated, the gravel materials had higher stresses based on the relatively rigid elastic modulus and resistance to deformation. Review of the stress contours indicated a small degree of buckling caused by the unbalanced load and heterogeneity of the soil and rock structure. These stresses however were too low to warrant any design changes to the frozen earth structure. Elastic displacements were computed to range from 0.5 to 0.8 feet (0.15 to 0.24 meters) radially.5. Thermal Design
For a project of this magnitude, it is essential that a thermal analysis is done during the design phase as well as during the execution of the work. Geo-Slope International's Finite Element Program TEMP/W was used for the thermal analyses. During the design phase, the thermal analyses provided maximum freeze pipe spacing to form a frozen wall in a given time in accordance with the structural design, required refrigeration capacity, and temperature distribution at any given time. During the execution phase, the thermal design served as a QA/QC tool to ensure and verify that the ground is actually frozen in accordance with the structural and thermal design submittals. The following thermal analyses deal with the execution part of the work. The as-built drawings as well as the actual measured brine temperatures in the field were used as input data and to model the FEM. The FEM model was then calibrated by using the actual measured data in the temperature pipes with the predictions of the FEM. After verification of a close match between measured and FEM predicted data, the FEM model could then be used to predict temperature distribution as well as freeze wall thickness at any time.| Input Data for Thermal FEM | Soil Stratum | ||
|---|---|---|---|
| II | III | ||
| Description | Units | Clay (CL) | Sand & Gravel w/ Boulders & Clay (S & G) |
| Saturated Density γs | PCF | 116.3 | 125 |
| g/cm³ | 1.86 | 2.01 | |
| Dry Density γd | PCF | 83.1 | 100.6 |
| g/cm³ | 1.33 | 1.61 | |
| Density Index G | - | 2.85 | 2.70 |
| Saturation Sr | - | 1.0 | 1.0 |
| Water Content w | % | 40.0 | 25.0 |
| Porosity n | % | 53.3 | 40.3 |
| Unfrozen Thermal Conductivity ku | BTU/(Ft Hr °F) | 0.66 | 0.89 |
| W/(m K) | 1.14 | 1.54 | |
| Frozen Thermal Conductivity kf | BTU/(Ft Hr °F) | 1.38 | 1.56 |
| W/(m K) | 2.39 | 2.70 | |
| Unfrozen Heat Capacity cu | BTU/(CF °F) | 50.04 | 45.42 |
| kJ/(m³ K) | 3356 | 3047 | |
| Frozen Heat Capacity cf | BTU/(CF °F) | 33.36 | 32.81 |
| kJ/(m³ K) | 2237 | 2200 | |
| Initial Soil Temperature | °F | 53 | 53 |
| °C | 11.7 | 11.7 | |
Table 5: Input Data for the Thermal Analyses
The thermal input data for the FEM are summarized in Table 5. The freeze pipe temperatures with time were calculated using the actual brine temperatures. Thermal analyses were performed in the four identified Strata of Table 1 and at various, strategic locations. Only the critical soft clays (Stratum II) are addressed in this paper. The best example – it includes the largest freeze pipe spacing - is a location on the West-side of the freeze wall (Fig. 1), which incorporates an external temperature monitoring pipe E-123A and an internal temperature monitoring pipe C-088A. The temperature sensor that monitors the soft clays (Stratum II) is at 40' (12.20 m) depth. The as-built locations of the freeze pipes and temperature pipes are shown in Fig. 8.
Fig. 8: As-Built Pipe Layout at 40' (12.20 m Depth)
Fig. 9 shows the comparison of the actual measured field data and the FEM prediction for the internal temperature monitoring pipe C-088A. As can be seen, the FEM prediction matches closely the actual field data; similar results were obtained for the external temperature monitoring pipe E-123A. This validates the FEM model for the prediction of the freezing progress in the clays.
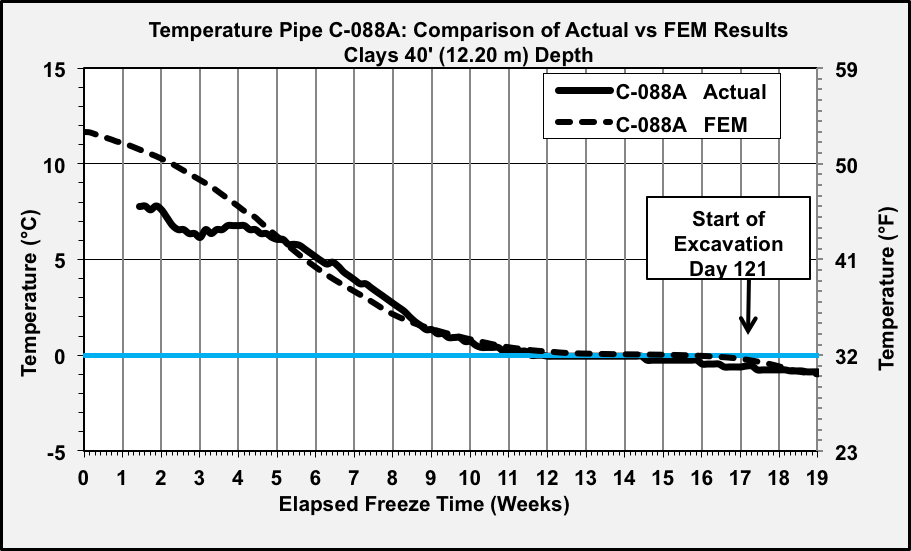
Fig. 9: Temperature Pipe C-088A Actual versus FEM Prediction
After a freezing time of 121 days, the main excavation below the water table started. The FEM showed that at this time the freeze wall had a thickness of 29' (8.84 m), which was slightly less than the structurally required 30' (9.15 m). However, when the excavation reached the depth of 40' (12.20 m) after 150 days of freezing, the freeze wall had a thickness of 30.5' (9.30 m) and was considerably colder than before. The temperature distribution after 150 days of freezing is shown in Fig. 10.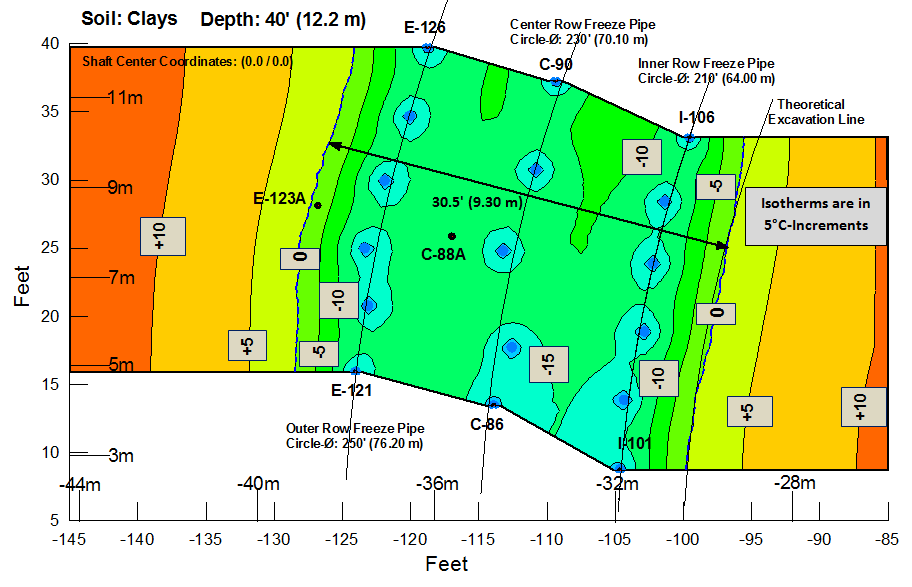
Fig. 10: Temperature Distribution after 150 Days of Freezing
